7 Sided Polygon Interior Angles
Interior Angles of Polygons
An Interior Angle is an angle inside a shape

Another example:

Triangles
The Interior Angles of a Triangle add together upwards to 180°
Let's try a triangle:
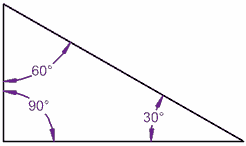
ninety° + lx° + 30° = 180°
It works for this triangle
Now tilt a line by 10°:
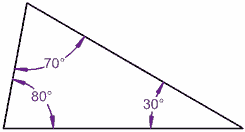
lxxx° + lxx° + xxx° = 180°
Information technology still works!
One angle went upward by 10°,
and the other went down by 10°
Quadrilaterals (Squares, etc)
(A Quadrilateral has 4 direct sides)
Allow's try a square:
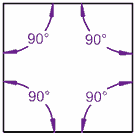
xc° + 90° + xc° + 90° = 360°
A Square adds up to 360°
Now tilt a line past ten°:
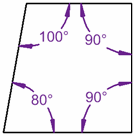
80° + 100° + 90° + xc° = 360°
Information technology still adds upward to 360°
The Interior Angles of a Quadrilateral add upwards to 360°
Because at that place are 2 triangles in a square ...
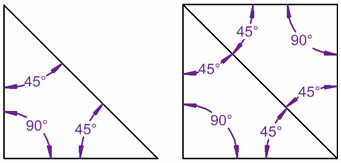
The interior angles in a triangle add together up to 180° ...
... and for the square they add up to 360° ...
... because the square can exist made from two triangles!
Pentagon
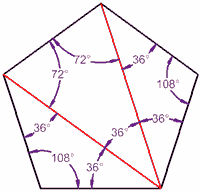
A pentagon has 5 sides, and tin can be fabricated from three triangles, and so you know what ...
... its interior angles add up to 3 × 180° = 540°
And when information technology is regular (all angles the aforementioned), then each angle is 540° / v = 108°
(Exercise: make certain each triangle here adds up to 180°, and check that the pentagon's interior angles add together up to 540°)
The Interior Angles of a Pentagon add together up to 540°
The General Rule
Each fourth dimension we add a side (triangle to quadrilateral, quadrilateral to pentagon, etc), we add together another 180° to the total:
So the general rule is:
Sum of Interior Angles = (n−2) × 180°
Each Angle (of a Regular Polygon) = (n−2) × 180° / north
Perhaps an instance volition assist:
Example: What near a Regular Decagon (10 sides) ?

Sum of Interior Angles = (north−two) × 180°
= (10−two) × 180°
= 8 × 180°
= 1440°
And for a Regular Decagon:
Each interior angle = 1440°/ten = 144°
Annotation: Interior Angles are sometimes called "Internal Angles"
7 Sided Polygon Interior Angles,
Source: https://www.mathsisfun.com/geometry/interior-angles-polygons.html
Posted by: brockmaneirdivemong.blogspot.com


0 Response to "7 Sided Polygon Interior Angles"
Post a Comment